We will show that the different versions of reality in the wave function are in separate universes which cannot affect each other in any way as time progresses. This result is demonstrated using a particular example, but the same arguments would hold whenever any ‘single-particle’ wave function (except perhaps that for a photon) simultaneously takes at least two different paths. It depends on the linearity of the theory.

Fire a spin silver atom into a Stern-Gerlach device. There will be one ‘trajectory’ traced out by the + wave function and another traced out by the – wave function. Let be the time-dependent three dimensional volume where the silver atom wave function is non-zero on the + trajectory and the corresponding volume on the – trajectory. These regions are well-defined even after the two branches of the silver atom wave function hit the detectors, and they are non-overlapping after the wave function of the silver atom clears the magnet.

Now consider the Schrödinger equation for the full wave function (including both versions of reality) describing the silver atom and the detectors;


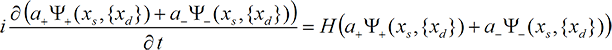
where the a’s give the relative sizes of the two versions, x_s is the coordinate of the silver ‘atom’ and { x_d } represents the coordinates of all the ‘atoms’ in the detectors (plus the observer if one wishes). denotes the branch of the wave function when x_s is in region , and the branch when x_s is in region . Because the two regions do not overlap, we have (assuming no long-range interactions for the silver atom)

(Note: The H in does not (substantially) change the location of the silver atom. Thus in Eq. (8A-2) is a function of x_s which has the value 0 when x_s is not in .)

Eqs. (8A-1), (8A-2) and (8A-3) imply the wave functions for the two branches obey their own separate equations of motion,


(and the Schrödinger equation is irrelevant (0=0) when x_s is in neither region.) Thus, because they obey their own separate equations, and because H is independent of the wave function in a linear theory, the evolution of is entirely independent of what happens on the branch (and vice versa). That is, the different versions act as if they were in different, non-communicating universes.

Further, we see that, once the two parts of the silver atom wave function have separated the coefficients a₊ and a_- can never change (in a unitary theory). See also Mathematical Collapse and Linearity on the need for non-linearity in mathematical collapse theories.